Mathematics is its own country within the continent of science. Unfortunately, it is just a bunch of islands. Where the capital island, i.e Number Theory, champions a fluent dialect of arithmetic and celebrates the monument of Primes, a tourist island (some call it Geometry) isolates with a rich culture of symmetry and transformations. These islands, rarely traversed between, stand at opposite ends of the nation... or they did, until the introduction of the Langlands program.
What is Langlands?
At its core, Langlands began as a venture connecting geometry to number theory by noticing and formalising unique similarities or parallels of both fields. This was an exciting endeavour for most mathematicians at the programme's conception, naturally arising from the interesting implications it brought. Imagine solving problems of neuroscience using orthopedy, or incorporating techniques of charcoal painting into Warli art. This was the weight of connecting geometry techniques to number theory and shook the modern mathematicians.
Without boring you with too much history, I will still provide a brief as to where Langlands originated. The programme was the brainchild of Robert Langlands in the text of a revolutionary letter he sent to André Weil. Commenting on mathematics' everlasting nature of its conjectures and theories that often stand unrefuted, Robert was one of the first to bring together critical mathematical conjectures that had grazed the surface of bridging geometry and number theory. He took inspiration from works of Harish-Chandra, Atle Selberg, Goro Shimura, and Hermann Weyl to present the interdisciplinary findings of these mathematicians. Oftentimes, such mathematicians are ex-physicians, or have switched fields giving them a comprehensive and intellectual oversight as to the connections that can be drawn.
Exploring an Example - Galois Groups and automorphism
Understanding a complex example at the core of the Langlands may be difficult for a math hobbyist like me, hence we can explore some of its simpler, peripheral problems.
Galois Groups are mathematical objects. Particularly, you must be aware of what polynomials are. For instance, we take a simple example of x² - 2 = 0. The roots of this equation are √2 and -√2. In a mathematical sense, these are considered symmetric. Notably, this symmetry is not geometrical where there is a "line" of symmetry, rather an abstract symmetry.
Why does this matter? Well, we know there are formulas for polynomials upto and including degree 4. You could solve these polynomial equations without any uncertainty, however, there is no formula for polynomials higher than degree 5! How do we solve them?By exploiting this very symmetry. And how do we do that? Langlands says functional analysis.
We just explored a topic of number theory, thus in the spirit of Langlands, we switch over to functional analysis to see automorphism. Now, what's that? Take a function like sin(x). What happens when you "morph" the input by adding 2π? Nothing, because sin(x+2π)=sin(x). This is automorphism. The ability of a function to retain form even after "morph"ing its input.
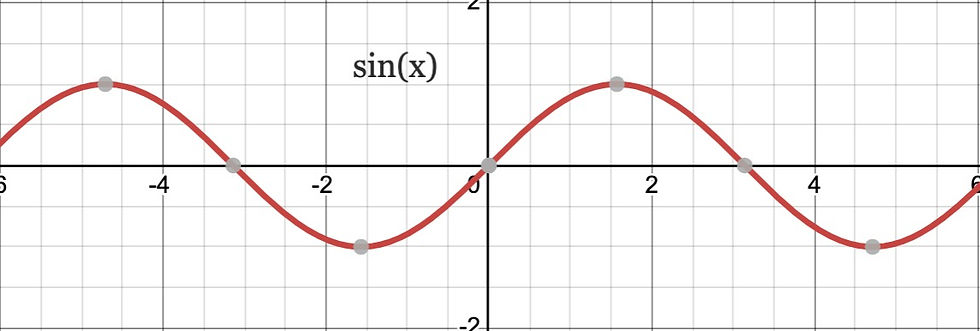
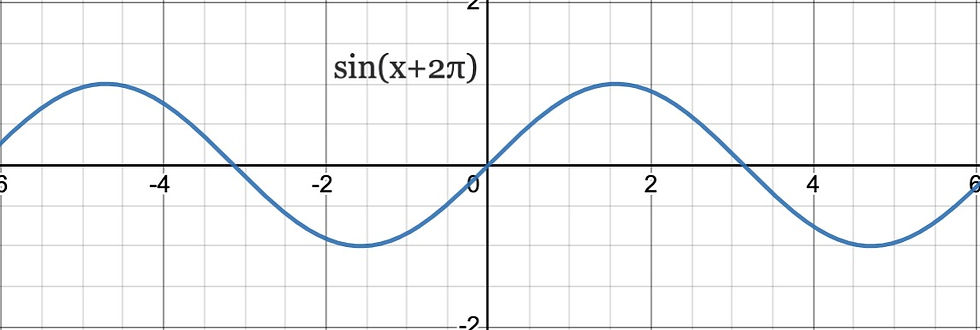
Now, imagine, Langlands managed to connect this property of functional automorphism to the challenging task of polynomial solving beyond degree 5. These were the bridges Langlands saw and Langlands formalised.
Future of Langlands
So far, Langlands has had its most famous application in Fermat's Last Theorem. Nonetheless, the future of Langlands is amusing for any mathematician. This is not due to its sheer curiosity generated and beautiful implications (although, that's reason enough), but also because of the innovative problems it introduces and/or solves. Recently, Peter Scholze had transformed the p-adic numbers into a geometric shape giving it new light and feasibility. Such observations open a possibility and direction of research unique to this century of mathematicians.
The Langlands programme is a must-explore for modern math enthusiasts due to its sheer infancy of research giving way to multiple avenues.
Bibliography and Suggested Further Reading
Datta, Sayantan. “Meet the Langlands Program, the World’s Biggest Maths Project | Explained.” The Hindu, 21 Nov. 2023, www.thehindu.com/sci-tech/science/what-is-the-langlands-program-explained/article67550905.ece. Accessed 29 Jan. 2024.
Kontorovich, Alex. “What Is the Langlands Program?” Quanta Magazine, June 2022, www.quantamagazine.org/what-is-the-langlands-program-20220601/.
Langlands, Robert. Handwritten Letter to André Weil. Jan. 1967, publications.ias.edu/sites/default/files/handwritten-ltw_rpl.pdf. Institute for Advanced Study.
Thomas, Kelly Devine. “Modern Mathematics and the Langlands Program - Ideas | Institute for Advanced Study.” Institute for Advanced Study, 2010, www.ias.edu/ideas/modern-mathematics-and-langlands-program.